Differential & Integral Calculus (lim, ∂, ∫) [Video Lectures]

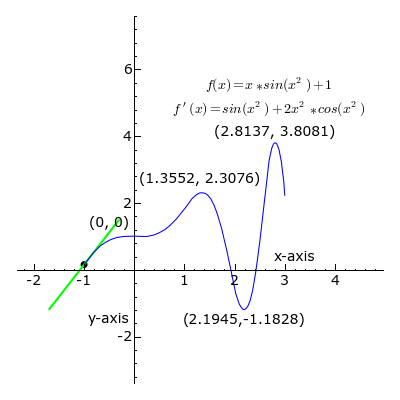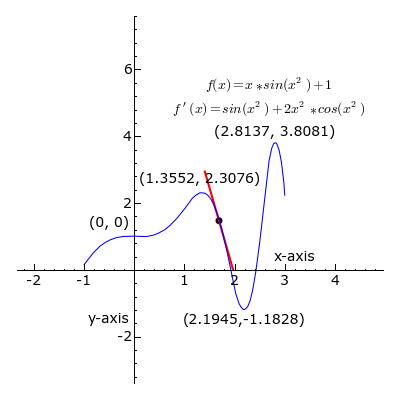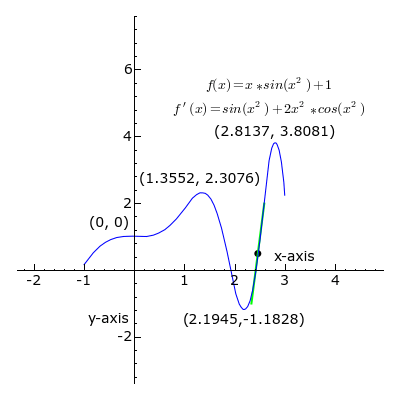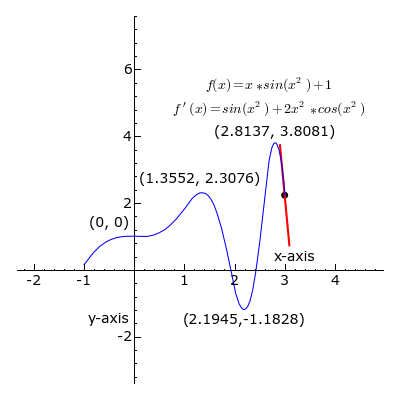
The Calculus is an important branch of mathematics. In this post we'll go through the lectures on differential and integral calculus, which are one of the hard problems students face during their college.

The "Derivative" is a measure of how a function changes as its input changes.Loosely speaking, a derivative can be thought of as how much one quantity is changing in response to changes in some other quantity; for example, the derivative of the position of a moving object with respect to time is the object's instantaneous velocity. Or how fast the temperature of a room is changing with respect to time. The derivative of a function at a chosen input value describes the best linear approximation of the function near that input value. For a real-valued function of a single real variable, the derivative at a point equals the slope of the tangent line to the graph of the function at that point. In higher dimensions, the derivative of a function at a point is a linear transformation called the linearization.


Understanding "Limits" is important before starting with derivatives or integration. Informally, a function f assigns an output f(x) to every input x. The function has a limit L at an input p if f(x) is "close" to L whenever x is "close" to p. In other words, f(x) becomes closer and closer to L as x moves closer and closer to p. More specifically, when f is applied to each input sufficiently close to p, the result is an output value that is arbitrarily close to L. If the inputs "close" to p are taken to values that are very different, the limit is said to not exist.

Above stuff seems confusing, let's take a deeper look at these concepts using the video lectures:



A Big Thanks to UCLA for posting these lectures.